Crystals are ubiquitous. Though they can give off a certain sense of luxury, crystals are found everywhere, from the salt sprinkled on our french fries to the snowflakes that melt on our tongues. Almost all solids are crystals, their atoms precisely arranged in regularly repeating patterns.

To a physicist, though, these everyday objects are profound. Somehow, atoms align into intricate and complicated repeating shapes, jungle gyms and honeycombs stretching out across space. From chaos, structure emerges. Crystals are the ultimate example of nature forming order where there was none before.

Crystals are defined by the fact that they are periodic, or regularly repeating, and form spontaneously. Imagine we take a glass of water and put it in the freezer. As the water cools, it will become ice, which is formed of repeating hexagons of water molecules. The water will crystallize all on its own—the process is spontaneous.

Just as crystals repeat in physical space, many phenomena repeat in time. For example, a drum sounds periodically in time as a drummer beats it. If this drum were to start beating spontaneously without the drummer, it could be called a time crystal. Just like a crystal in physical space, a time crystal would be periodic in time and would repeat spontaneously.

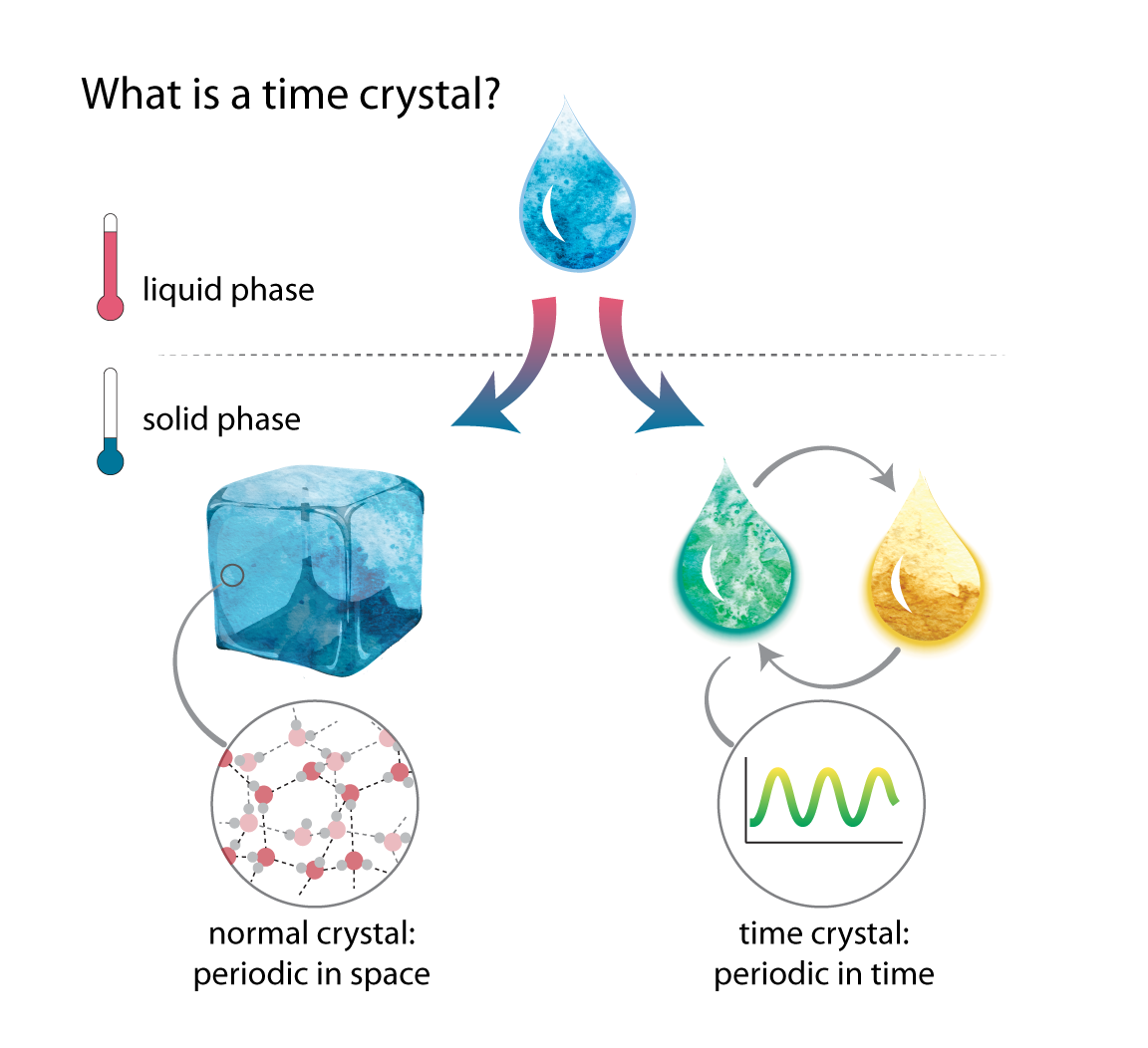As matter is cooled and undergoes a phase transition, a crystal can form spontaneously and is periodic in space. A time crystal would similarly become periodic in time.

Of course, a drum can’t keep on making sound without a drummer. But what about atoms—can they somehow spontaneously repeat themselves in time?

When time crystals were first proposed in 2012, they were immediately controversial, seeming somehow natural, yet impossible. Over the next five years, researchers at UC Berkeley and around the world unraveled the puzzle of time crystals, showing how and when they would—and would not—exist.

When a symmetry shatters

Crystallization is rooted in a deep phenomenon known as spontaneous symmetry breaking.

It’s pretty common for physicists to get worked up about symmetry, and for good reason—besides being mathematically beautiful, the concept of symmetry allows physicists to classify matter into phases. The everyday phases of matter are gas, liquid, and solid, where matter becomes more and more rigid. But these are hardly all of the possible phases of matter. Some phases are magnetic, and some are not; some can be compressed, and some cannot; some conduct electricity, some insulate, and some even superconduct. By and large, these various phases can be differentiated by how symmetrical they are, and which particular symmetries they have.

We are all familiar with the idea of symmetry, seen in the lines dividing Leonardo da Vinci’s Vitruvian Man. Symmetry is a property of an object that is unaffected by certain kinds of changes. For instance, a sphere is symmetric under rotations—any way you turn a uniformly colored marble, it looks exactly the same. A cube is also symmetric under rotations, but only by right angles—a weaker symmetry.

The laws of physics—the equations that tell matter how to move—can also have symmetries. For instance, the law of gravity tells a ball to roll downhill. This is true anywhere on the planet: if we take a flight from Berkeley to Uruguay, we will find that a ball will still roll downhill. Shifting from Berkeley to Uruguay didn’t do anything to the law of gravity, so we say that gravity is symmetric under shifting positions. The law itself is symmetrical.

Symmetries in the laws of physics almost always lead to symmetries in the matter that they describe. For instance, rain droplets reflect the symmetries of the law of gravity: they are symmetric around the downward axis because gravity doesn’t change when you rotate about that axis. Similarly, a balloon filled with hot gas will remain spherical as it expands, because the laws governing the balloon’s expansion don’t pick out any particular direction. It seems counterintuitive that rain or balloons would be asymmetrical, since the laws of physics are telling them to form in a symmetric way.

But symmetry itself isn’t a law. It can be broken, something physicists call spontaneous symmetry breaking. Rain could, in theory, fall in horizontal needles, or our balloon could expand unevenly to look like a hotdog. Strange as it sounds, spontaneous symmetry breaking is at the heart of some of our most important physical theories.

Crystallization, in fact, is the ultimate example of spontaneous symmetry breaking in nature. Norman Yao, assistant professor of physics at UC Berkeley, likes to explain it with a chessboard, which contains a regular grid of squares. “The idea of crystallization is somehow forming a spatial pattern on top of this chessboard,” he says. The chessboard looks exactly the same if we move all the squares by one to the right—”shifting by one square” is a symmetry. But a pattern on top of the board might have a different symmetry. For instance, if we pile sand on every other square, the sand piles would only be symmetric under shifting by two squares. They have a different symmetry from the chessboard, so the symmetry of the chessboard is broken.

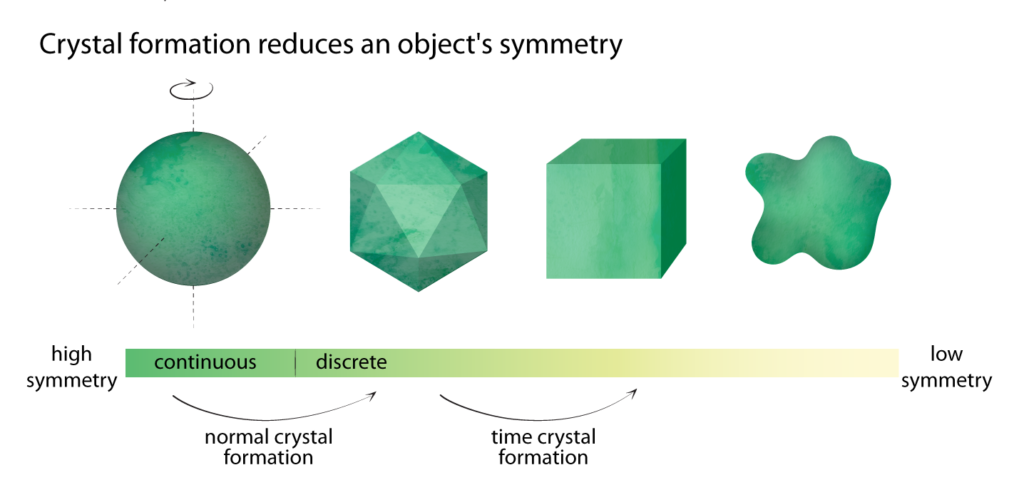The symmetry in the spatial arrangement of atoms in a normal crystal, or the temporal beating of a time crystal, changes during a phase transition.

One common symmetry of the laws of physics is that of time translation symmetry, which means that the laws of physics don’t change with time. Gravity, for instance, does not change over time—it doesn’t suddenly get stronger or weaker.

However, think of a musician beating a drum. Since the drum is being struck regularly by an outside force, from the drum’s perspective, the laws of physics do change with time—its equations change. Every so often, the drumstick tells the drum to beat, and the forces on the drum suddenly get much stronger.

Without a drummer, though, the laws do not change with time. Since there is no outside force, the drum just sits quietly. For time translation symmetry to be broken, the unstruck drum would have to somehow start to beat all on its own.

This is a wild idea. Yet crystals are not all that different—nothing is telling the atoms of a crystal to form a particular pattern, but nonetheless they start to form one on their own. After Nobel Laureate and physicist Frank Wilczek postulated the existence of time crystals in 2012, the world of physics was on edge. Could time crystals actually exist?

When UC Berkeley physics graduate student Haruki Watanabe began to tackle the problem, the answer appeared to simply be no.

Why there are no time crystals

Physicists were suspicious of Wilczek’s proposal, and with good reason. Quantum mechanics is governed by Schrödinger’s equation—of feline fame—and Schrödinger’s equation treats time very differently than space.

Schrödinger’s equation is the central law of quantum mechanics. It governs atoms, electrons, and the very small, just as Newton’s laws govern planets, apples, and the everyday. Crucially, it draws a distinction between those things that can be measured and those that cannot. Distances between atoms can be directly measured, but time cannot. Clocks, for instance, measure time only by measuring distances between hands and markings directly. Since time is so different from space in quantum mechanics, the argument goes, we shouldn’t expect the idea of a crystal to carry over.

Wilczek’s proposed time crystal would have to be periodic, or regularly repeating, in its calmest, lowest-energy configuration, referred to in quantum mechanics as the ground state. For instance, imagine a tetherball tied to its pole. A tetherball’s ground state is just to hang motionless, since this has the lowest energy. In contrast, a wildly swinging tetherball would be have high energy. Now, if this tetherball were a time crystal, its lowest energy state would be to swing around at the same slow speed, forever; bizarrely, it couldn’t sit still.

Physicists pushed back on Wilczek’s proposal from the beginning. In a matter of months, a flaw in Wilczek’s paper was found by Patrick Bruno, a physicist at the European Synchrotron Radiation Facility in Grenoble, France. While his initial example was wrong, Wilczek countered, time crystals might still exist elsewhere—Bruno hadn’t proven time crystals couldn’t exist. A heated back and forth ensued, with no clear victor.

Back at UC Berkeley, Watanabe became fascinated by Wilczek’s time crystal concept. Watanabe had made strides on spontaneous symmetry breaking in his first research papers, and in his qualifying exam he was peppered with questions about the possibility of time crystals. “I couldn’t answer these questions during the exam,” he recalls. “But I found it an interesting problem and kept thinking about it, and realized there must be something wrong in the original proposal.”

To Watanabe, Wilczek’s time crystal seemed impossible. Stationary objects always have lower energy than moving objects; how could motion exist in the ground state at all? “It’s literally a perpetual motion machine,” he says. “It would be very weird if such a state existed.”

To disprove Wilczek once and for all, Watanabe, together with Professor Masaki Oshikawa of the University of Tokyo, turned to a bread-and-butter concept in condensed matter physics: correlation. If two atoms can influence one another, they are correlated with a certain strength, while if they are independent, they are uncorrelated.

Watanabe and Oshikawa’s argument relied on the following definition: in a time crystal, spatially distant points must regularly swing from correlated to uncorrelated and back again. Using technical arguments, they were able to show that, for a ground state, such a correlation pattern could never exist. Time crystals were, simply, impossible.

Time crystals, though a tantalizing idea, seemed to have run out of road. That was, at least, until a few years later.

The jump rope loophole

In the summer of 2016, two theoretical research groups—one led by Professor Chetan Nayak at the University of California, Santa Barbara, and the other by Professor Shivaji Sondhi at Princeton University—realized that there was a loophole in the time crystal impossibility argument. The key to breaking time translation symmetry, they claimed, was to first weaken it. They imagined collections of atoms that, like a drum being beaten, were regularly jolted.

For the atoms, the laws of physics were only time translation symmetric by a discrete amount, the time between the jolts. This is the distinguishing feature of a periodically driven system: time translation symmetry is present, but weaker. Breaking this weaker symmetry would look a bit like the pattern on top of the chessboard, or like a drum that only sounded on every other hit.

“Imagine the two of us are playing jump rope,” says Yao. As we swing our arms, he explains, the jump rope rises and falls regularly. Typically, a jumper would just jump once for every swing, but if the jumper decided to do double-unders, the rope would go around twice for every jump. The jumper would then break the symmetry of the jump rope. “Somehow it doesn’t feel natural,” says

Yao. “But that’s the essence: that the system’s action is now at a different period than the driving.” They dubbed the phenomenon a discrete time crystal, a discretized version of Wilczek’s proposal.

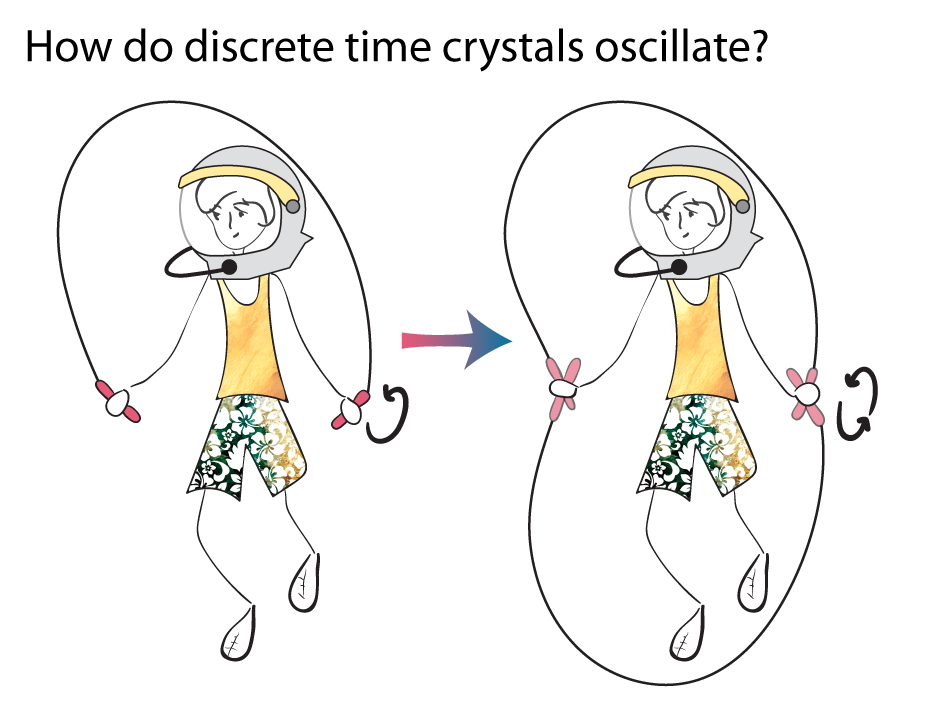As a time crystal forms, the frequency of its oscillations decreases. Like a jumper responding to the beat of the jump rope, the jumper goes from jumping once per swing to once every other swing.

When such a phenomenon happens in a normal crystal—when the chessboard builds up sand piles only on every other square—it is called a density wave. Strictly speaking, what the Princeton and UCSB groups had discovered was a time density wave—a pattern of oscillations that could build up on top of the regular jolting in time. To Watanabe and Oshikawa, the term discrete time crystal did not fit this weaker phenomenon, but by now, the name had stuck.

Staying cool and sticking together

There was, however, one enormous problem with the loophole: heating. Since we are jolting the atoms and giving them energy, they should eventually get hotter and hotter, until nothing is left but a featureless soup—a heat death. This featureless soup couldn’t keep responding to only every other drumbeat, and would eventually lose its time crystal property.

Luckily, a quantum strategy for preventing this thermalization had recently been discovered, deemed many body localization.

Quantum mechanics gives striking predictions about systems with disorder, or inherent randomness. Despite the regularity of a crystal, there is still some wiggle room—each atom may be randomly jiggling off its perfect spot, and there may even be the occasional atom missing or extra speck of dirt. Say that we froze a bucket of water with some dust particles floating around that get trapped inside the ice crystals. Many body localization predicts that the water’s electrons will tend to stick to the specks of dust. Like flies stuck to pots of honey, no matter how they’re pushed and cajoled by outside forces, nothing can unstick the electrons from their dusty hosts. Heating causes electrons to move around faster as they reach higher temperatures, but since localized electrons are immobile, they can’t heat up.

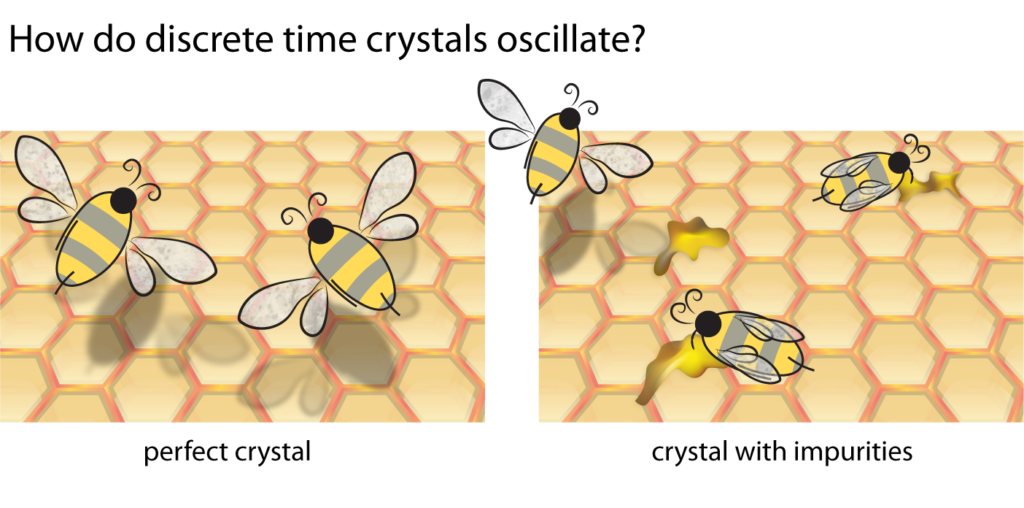Like bees attracted to honey on a honeycomb, electrons like to stick (“localize”) to the impurities in crystals. This locks electrons in place, keeping a time crystal cool.

Localization was not the only escape route from heat death, however. Another proposal of the UCSB group was to use the phenomenon of prethermalization, that is, the tendency of some quantum systems to take an incredibly long time to heat up. Eventually they will get hot and melt, but the time required may be much longer than the age of the universe—and importantly, that could never be seen in a realistic experiment. For all intents and purposes, these slow-heaters would be time crystals in just the same way as a true localized system.

Without a way to avoid heating, discrete time crystals simply could not exist. Like ice left too long in the sun, the time crystal would melt, losing its rigid order. Localization or prethermalization, it turns out, are essential ingredients in the time crystal formula.

With these ingredients in place, the real test was to observe discrete time crystals in the lab—to prove they weren’t just theoretical ramblings.

Riding the time density wave

But how would one even know if one had, in fact, created a time crystal? What would distinguish it uniquely in an experiment?

Yao, along with former UC Berkeley colleagues Andrew Potter, Ionut-Dragos Potirniche and Ashvin Vishwanath, had an answer.

“We started to ask a very simple question,” recalls Yao. “What is the smoking gun experimental signature of a time crystal?” Their answer was in their rigidity, or robustness to making mistakes. Any imperfections in the drum beats—if the drummer was late sometimes, or early other times—just didn’t matter to a time crystal. Remarkably, the response was always the same.

Following their initial proposal, Yao worked closely with two experimental groups to realize the time crystal phase: Professor Chris Monroe’s group at the University of Maryland, and his old advisor Professor Misha Lukin’s group at Harvard.

Each experiment took a different track in searching for this signature of time crystals. The Maryland group tried to emulate the simple theoretical models as closely as possible using ultra-cold atoms, a platform with extremely precise control. Lukin’s group, on the other hand, used a different platform for their experiments: that of nitrogen vacancy centers. In this platform, a piece of diamond is created with a specific type of defect—namely, two central carbons are replaced by a single nitrogen and a hole, or a missing carbon, to which the nitrogen atom is bonded.

Neither experiment was perfect, but their harmonious observation of rigid period doubling provided strong evidence for the observation of a time crystal. The jump rope phenomenon is possible. The two experiments were jointly published in March 2017 as the first evidence of this nascent nonequilibrium phase of matter.

Time crystals: gateways out of equilibrium

Time crystals have proven to be a rich and exciting physical phenomenon—one thought impossible until only recently. However, their greatest promise may lie in the general study of matter out of equilibrium, where physicists are just getting their toes wet.

Physicists have developed a deep understanding of systems in equilibrium from centuries of research into thermodynamics. For the laws of thermodynamics to apply, though, sudden changes are forbidden. Thermodynamics says nothing about systems that are jolted or shaken suddenly out of their calm equilibrium states. For many processes in nature, equilibrium thermodynamics doesn’t apply, and new tools have to be developed.

Periodically driven systems are in some sense the easiest out-of-equilibrium systems to study, and understanding them could be the key to unlocking vast, uncharted physics territory.

“Time crystals are the ‘gateway drug’—they’re showing us that there’s interesting stuff to think about in periodically driven systems,” says Yao, smiling. “We’re just reaching the tip of the iceberg.”

Will Berdanier is a graduate student in physics.

Feature image credit: Nicole Repina

This article is part of the Fall 2017 issue.